Chaos on a string
There is an enormous pendulum hanging in the Science LinX exhibition. You can do neat tricks with it. Make it swing back and forth... Is that it? No. This pendulum is different. It’s totally unpredictable. Pure chaos. Chaos on a string. And that’s cool. (At least we think it is!)

Unpredictable
Our chaotic pendulum is at first glance just your average pendulum. A long chain with a weight hanging on it, similar to the pendulum in an old-fashioned grandfather clock. These pendulums oscillate, i.e. they move back and forth in a regular rhythm. Give the pendulum a push and it will swing back and forth in a regular motion until it runs out of momentum. Our pendulum, however, swings entirely irregularly and unpredictably. The motion of the Science LinX pendulum can be described by a so-called ‘strange attractor’: a mathematical model of a dynamic system that never displays the same pattern more than once because of its complex motion.

Minor causes lead to major consequences
If you were to push our pendulum ten times using the same amount of force, you would find that it followed ten different oscillations. This has everything to do with the first push which, although similar to subsequent pushes, is never exactly the same. There will always be tiny differences between the pushes (you might push just that little bit harder, or in a slightly different direction). These tiny differences at the start have large consequences for the pendulum’s motion. This is typical of chaos: acute sensitivity to initial conditions. In other words, ‘minor causes, major consequences’. Another characteristic of chaos is that its path is difficult to predict. This is the consequence of multiple non-linear forces that are simultaneously exerted on the system. This unpredictability increases over time. To recap: minor causes, major consequences. In the case of the pendulum, you can imagine it would take quite some time before two pendulums with nearly identical starting conditions eventually display entirely distinct patterns.
Chaotic climate model
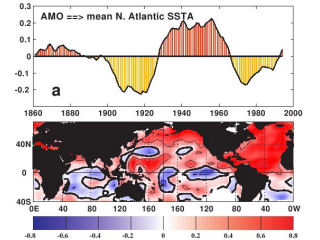
Although our pendulum may well display chaotic behaviour, never repeat the same pattern and be unpredictable, on paper we’ve got it completely under control! Using mathematical models we can describe complex dynamic systems accurately and so we understand them better. Groningen’s Dynamic Systems research group, led by Henk Broer, specializes in constructing and interpreting these models. Complicated mathematical models are used here to improve our understanding of our physical reality. Whether this concerns climatological, biological or physical processes, we can use the same maths to describe them. At present, Groningen PhD student Alef Sterk is working on a climate model for the North Atlantic Multidecadal Oscillation. He is interested in the slow fluctuations in water temperature in the Atlantic Ocean and how this relates to precipitation levels in Europe, for example. Sometime ago, the same maths was used in the Centre for Ecological and Evolutionary Studies (CEES) to describe an entirely different fluctuation...
Chaotic ecosystem
Biologists have been trying to understand the plankton paradox for years: the fact that dozens of species of plankton can survive alongside each other in an environment that offers only a handful of different sources of food. You would expect all these different species to get in each other’s way. A species with specialized feeding habits would be expected to outcompete the other species. Eventually, you would expect about as many species of plankton as there are sources of food. The actual situation, however, is quite different. How are so many different species able to coexist? Here, too, it was the chaos theory that provided the answer.
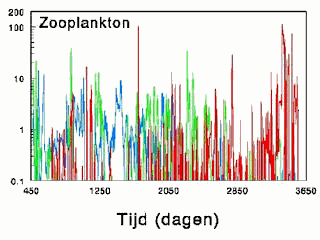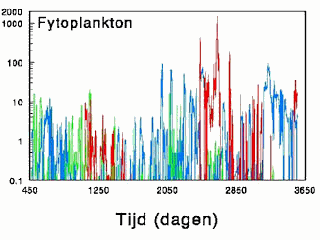
Research in Groningen
Two of Groningen’s theoretical biologists, Franjo Weissing and Jef Huisman, demonstrated that a chaos effect sets in as soon as species compete for three or more different sources of food. Different species of plankton compete with each other in different ways and at different rates. This leads to population fluctuations, so that no one species can ever permanently dominate the others. This creates space, albeit a predefined space, as the limited number of food sources prevents fluctuation from getting out of hand. And here we have a typical characteristic of chaos: a continuously changing pattern (the ‘dominant’ species continually changes) within a predefined space. Moreover, by carrying out a wide range of simulations, Weissing and Huisman demonstrated that this species dynamism is strongly dependent on initial conditions! This is typical of a chaotic system. Plankton ecosystems that initially display the same species variation slowly develop in different directions until they eventually become completely unalike. In other words, their pendulums were pushed in another direction!
Links
Acknowledgements
Special thanks to: Hüttinger Exhibition Engineering, Charlotte Vlek and Lenny Taelman. Please contact Science LinX if you should have been included in the acknowledgements.
Author
Siëlle Gramser
| Last modified: | 23 December 2016 2.15 p.m. |

